درس 3: اعمال جبری روی توابع
ریاضی (2)
یازدهم
دوره دوم متوسطه- نظری
علوم تجربی
درسنامه آموزشی این مبحث
اگر $f(x)=\left\{ \begin{matrix} 2x,x\lt 1 \\ x,x\ge 1 \\ \end{matrix} \right.$ و $g(x)=\left\{ \begin{matrix} 4x,x\lt 2 \\ 3x,x\ge 2 \\ \end{matrix} \right.$، ضابطهٔ تابع $y=f(x)-g(x)$ کدام است؟
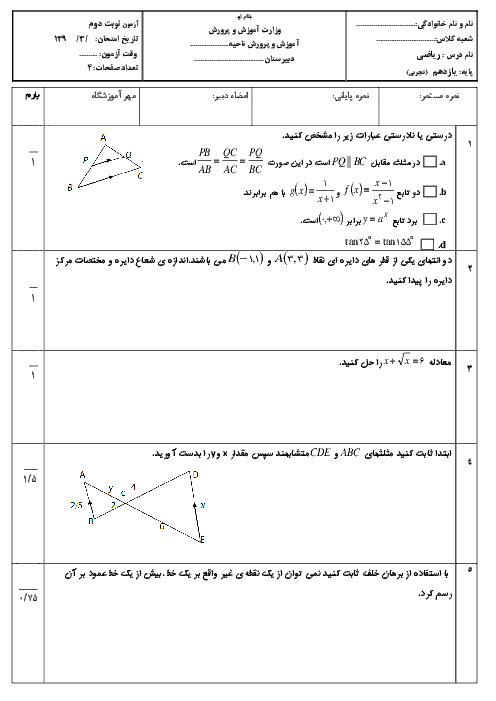
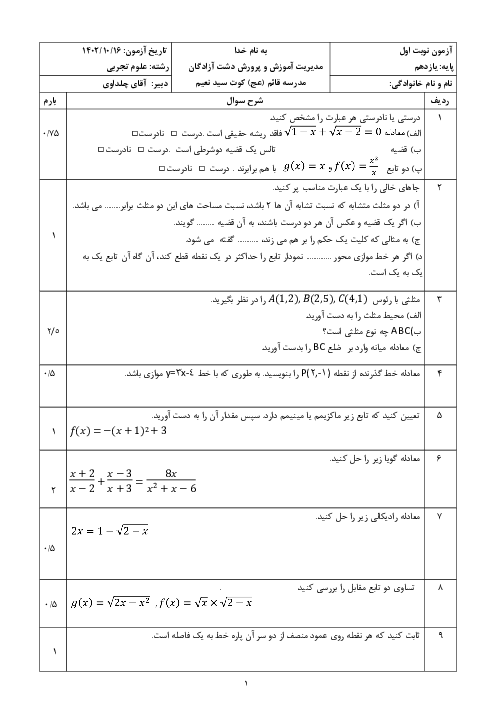
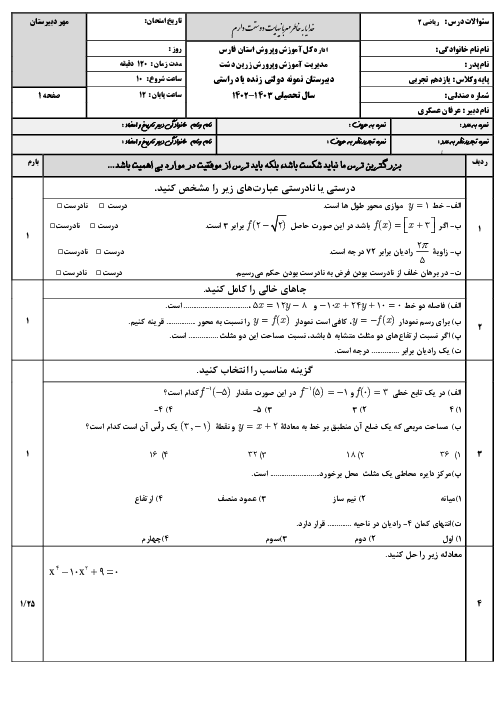
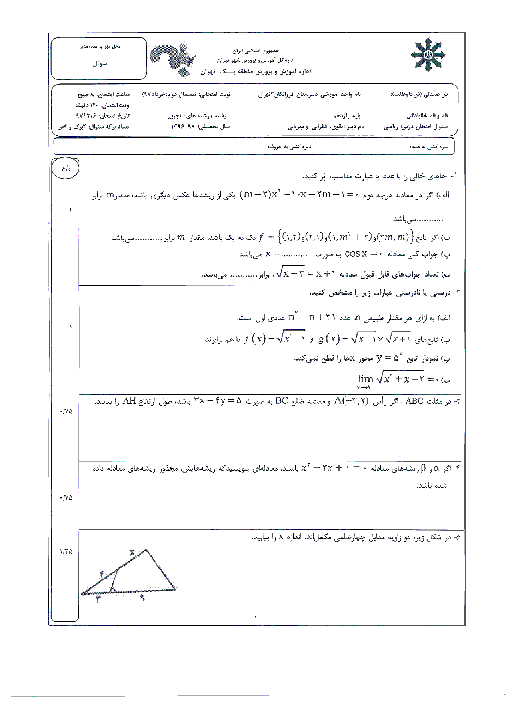
1 )
$y=\left\{ \begin{matrix} -2x,x\lt 1 \\ -3x,1\le x\lt 2 \\ -x,x\ge 2 \\ \end{matrix} \right.$
2 )
$y=\left\{ \begin{matrix} -x,x\lt 1 \\ -3x,1\le x\lt 2 \\ -2x,x\ge 2 \\ \end{matrix} \right.$
3 )
$y=\left\{ \begin{matrix} 6x,x\lt 1 \\ 4x,x\ge 2 \\ \end{matrix} \right.$
$y=\left\{ \begin{matrix} -2x,x\lt 1,x\ge 2 \\ -3x,1\le x\lt 2 \\ \end{matrix} \right.$
پاسخ تشریحی :
تحلیل ویدئویی تست
عباس ابراهیمی علویجه 
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...