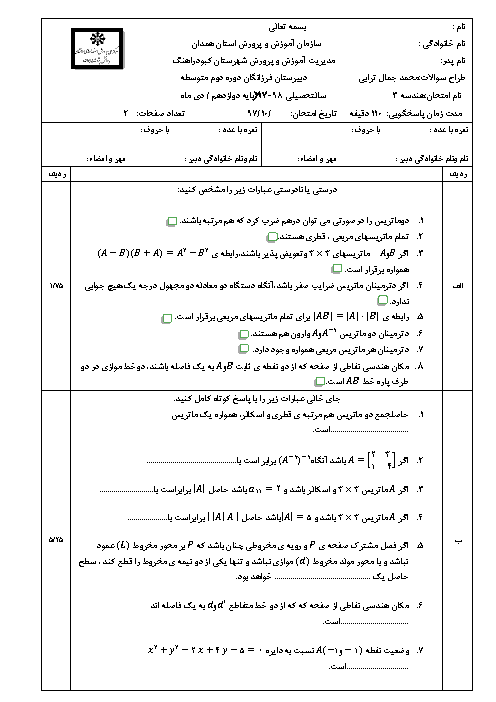
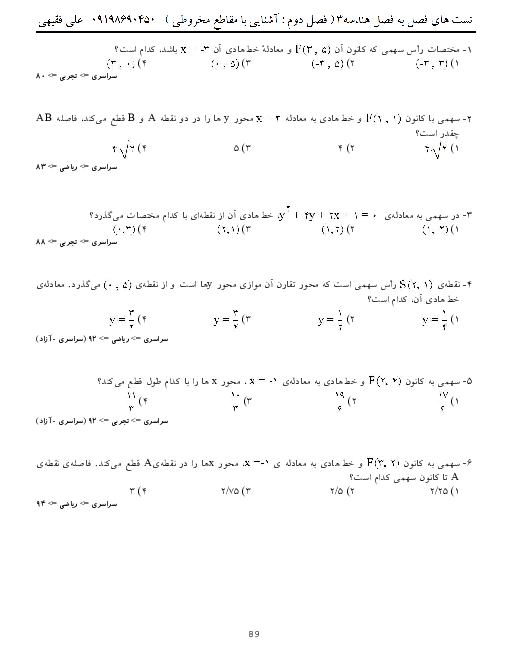
نکته: اگر $A$ ماتریسی مربعی باشد، توان‌های $A$ به‌صورت ${{A}^{2}}=A\times A$، ${{A}^{3}}=A\times {{A}^{2}}$، ... و ${{A}^{n}}=A\times {{A}^{n-1}}$ تعریف می‌شود.

نکته: ${{\left[ \begin{matrix} \begin{matrix} a & 0  \\ \end{matrix}  \\ \begin{matrix} 0 & b  \\ \end{matrix}  \\ \end{matrix} \right]}^{n}}=\left[ \begin{matrix} \begin{matrix} {{a}^{n}} & 0  \\ \end{matrix}  \\ \begin{matrix} 0 & {{b}^{n}}  \\ \end{matrix}  \\ \end{matrix} \right]$

با استفاده از نکات بالا داریم:

${{A}^{2}}=\left[ \begin{matrix} \begin{matrix} 4  \\ -2  \\ \end{matrix} & \begin{matrix} 6  \\ -4  \\ \end{matrix}  \\ \end{matrix} \right]\left[ \begin{matrix} \begin{matrix} 4  \\ -2  \\ \end{matrix} & \begin{matrix} 6  \\ -4  \\ \end{matrix}  \\ \end{matrix} \right]=\left[ \begin{matrix} \begin{matrix} 4  \\ 0  \\ \end{matrix} & \begin{matrix} 0  \\ 4  \\ \end{matrix}  \\ \end{matrix} \right]$

${{A}^{6}}={{({{A}^{2}})}^{3}}={{\left[ \begin{matrix} \begin{matrix} 4  \\ 0  \\ \end{matrix} & \begin{matrix} 0  \\ 4  \\ \end{matrix}  \\ \end{matrix} \right]}^{3}}=$$\left[ \begin{matrix} \begin{matrix} {{4}^{3}} & 0  \\ \end{matrix}  \\ \begin{matrix} 0 & {{4}^{3}}  \\ \end{matrix}  \\ \end{matrix} \right]=\left[ \begin{matrix} \begin{matrix} 64  \\ 0  \\ \end{matrix} & \begin{matrix} 0  \\ 64  \\ \end{matrix}  \\ \end{matrix} \right]$

بنابراین مجموع درایه‌های ماتریس ${{A}^{6}}$ برابر است با: $64+0+0+64=128$