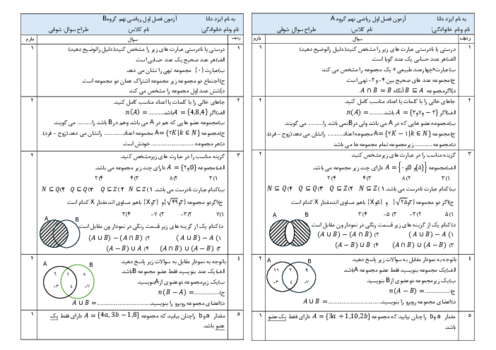
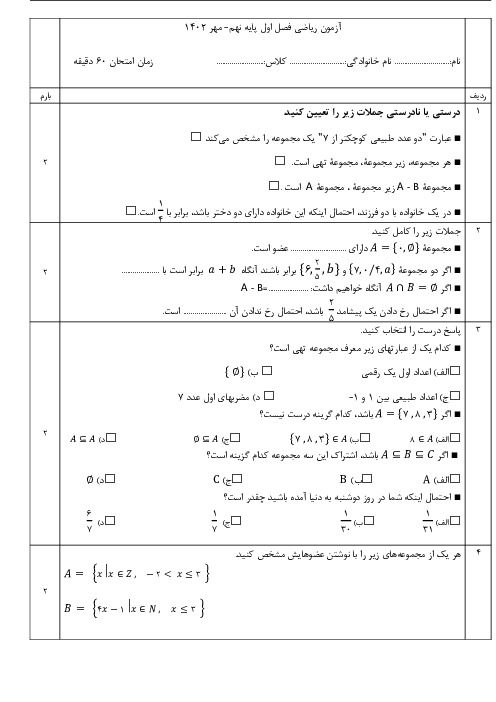
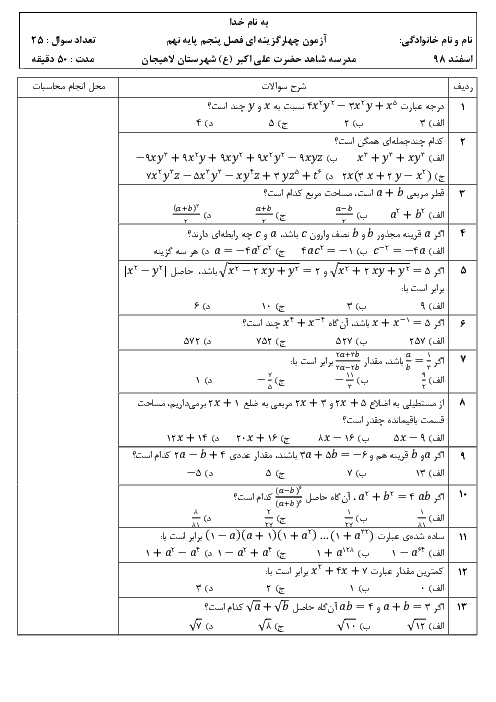
$S = \{ x \in \mathbb{R}|\mathop {\underbrace { - 5 \lt x \lt 5,{x^2} \in \mathbb{N}}_ \downarrow }\limits_{ - \sqrt {24} , - \sqrt {23} , - \sqrt {22} ,...,\sqrt {23} ,\sqrt {24} } \}  \Rightarrow n(s) = 48$

شمارنده‌های طبیعی 12: $\left\{ {1,2,3,4,6,12} \right\}$

$A = \{ \mathop {\sqrt 1 }\limits_{\mathop  \downarrow \limits_1 } ,\mathop {\sqrt 4 }\limits_{\mathop  \downarrow \limits_2 } ,\mathop {\mathop {\sqrt 9 }\limits_ \downarrow  }\limits_3 ,\mathop {\mathop {\sqrt {16} }\limits_ \downarrow  }\limits_4 \}  \Rightarrow P(A) = \frac{{n(A)}}{{n(S)}} = \frac{4}{{48}} = \frac{1}{{12}}$