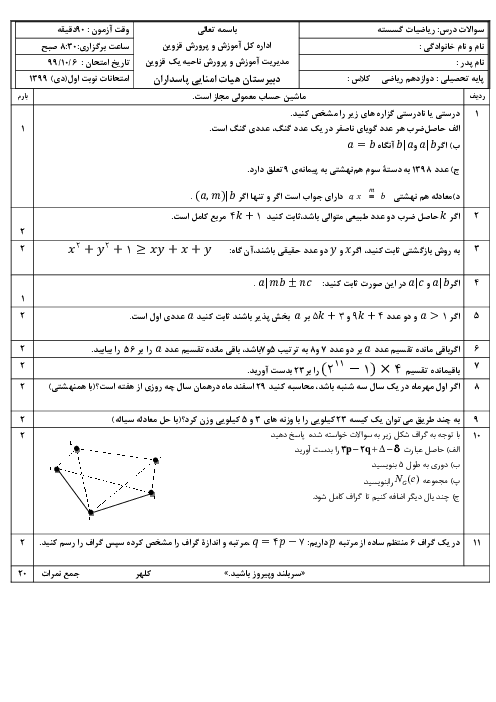
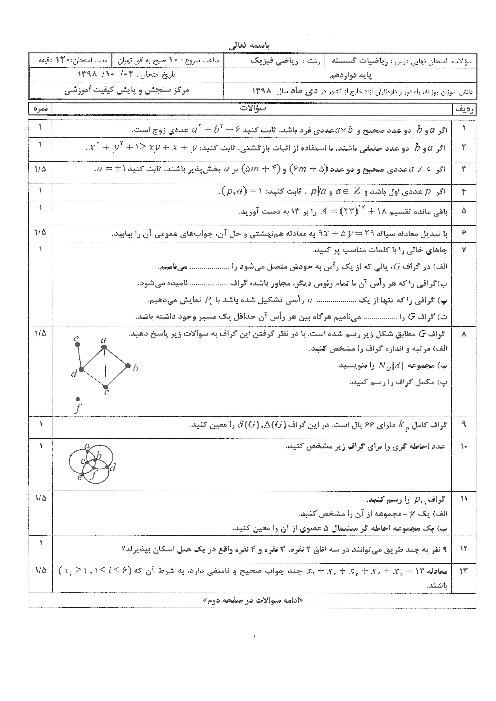
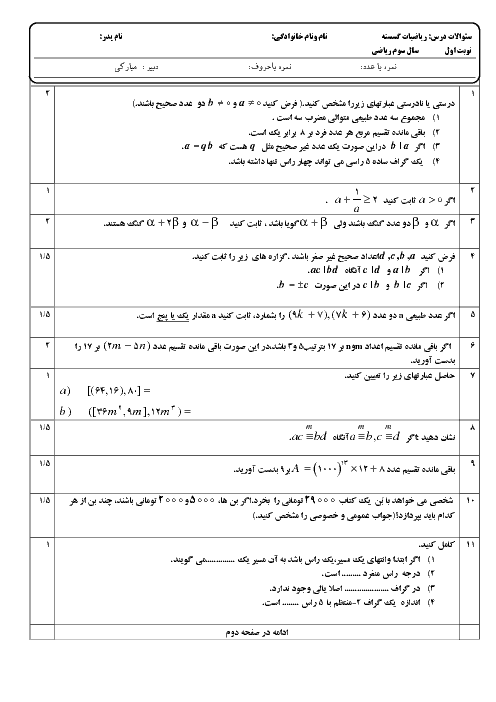
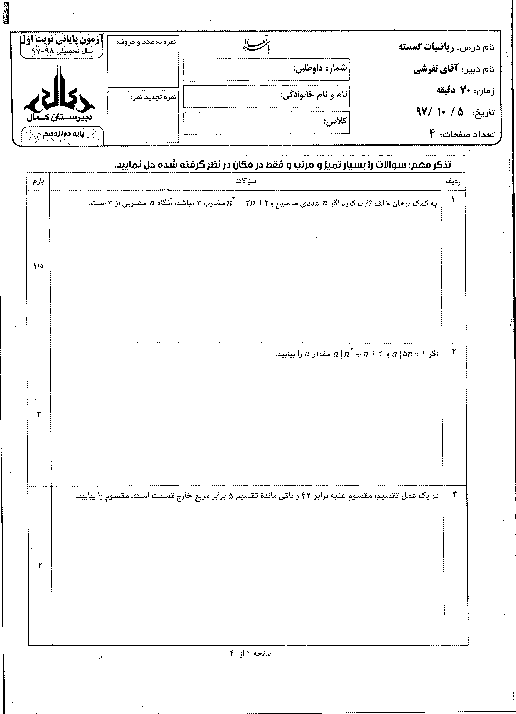
ابتدا باقی ماندهٔ تقسیم توان‌های 5 بر 31 به ترتیب حساب می‌کنیم تا به یک هم‌نهشتی مطلوب برسیم:

${{5}^{1}}\overset{31}{\mathop{=}}\,5,\,\,\,\,{{5}^{2}}\overset{31}{\mathop{=}}\,25\overset{31}{\mathop{=}}\,25-31\overset{31}{\mathop{=}}\,-6$

${{5}^{3}}\overset{31}{\mathop{=}}\,5\times (-6)\overset{31}{\mathop{=}}\,-30\overset{31}{\mathop{=}}\,-30+31\overset{31}{\mathop{=}}\,1$

اکنون می‌توان 

${{5}^{3}}\overset{31}{\mathop{=}}\,1\Rightarrow \left\{ \begin{align}  & {{({{5}^{3}})}^{2n+1}}\overset{31}{\mathop{=}}\,1\Rightarrow {{5}^{6n+3}}\overset{31}{\mathop{=}}\,1\Rightarrow 5\times {{5}^{6n+3}}\overset{31}{\mathop{=}}\,5 \\  & {{({{5}^{3}})}^{n}}\overset{31}{\mathop{=}}\,1\Rightarrow {{5}^{3n}}\overset{31}{\mathop{=}}\,1\Rightarrow {{5}^{2}}\times {{5}^{3n}}\overset{31}{\mathop{=}}\,{{5}^{2}} \\ \end{align} \right.$

$\left\{ \begin{align}  & {{5}^{6n+4}}\overset{13}{\mathop{=}}\,5 \\  & {{5}^{3n+2}}\overset{31}{\mathop{=}}\,25 \\ \end{align} \right.\Rightarrow {{5}^{6n+4}}+{{5}^{3n+2}}+1\overset{31}{\mathop{=}}\,5+25+1\overset{31}{\mathop{=}}\,31\overset{31}{\mathop{=}}\,0$

در نتیجه برای هر عدد طبیعی $n$، عبارت ${{5}^{6n+4}}+{{5}^{3n+2}}+1$ بر 31 بخش‌پذیر است.