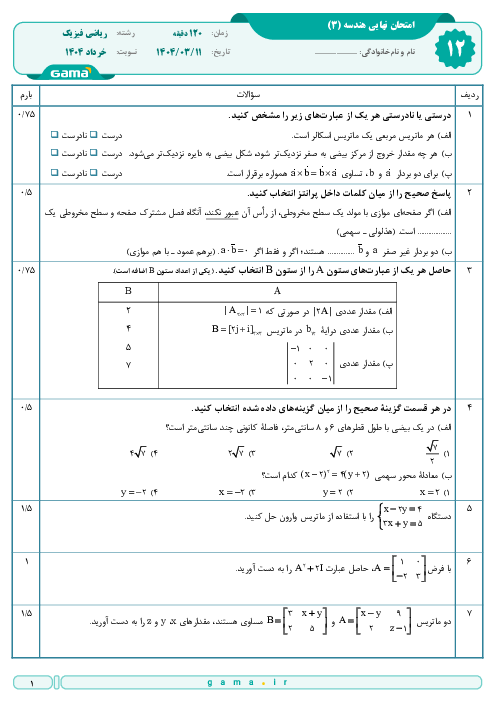
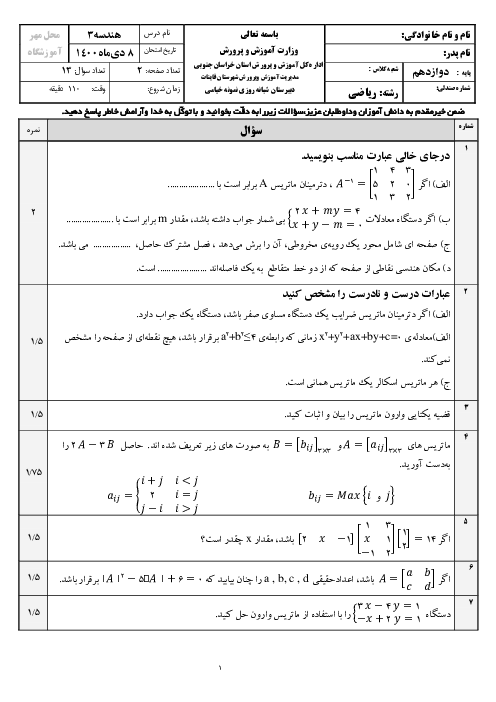
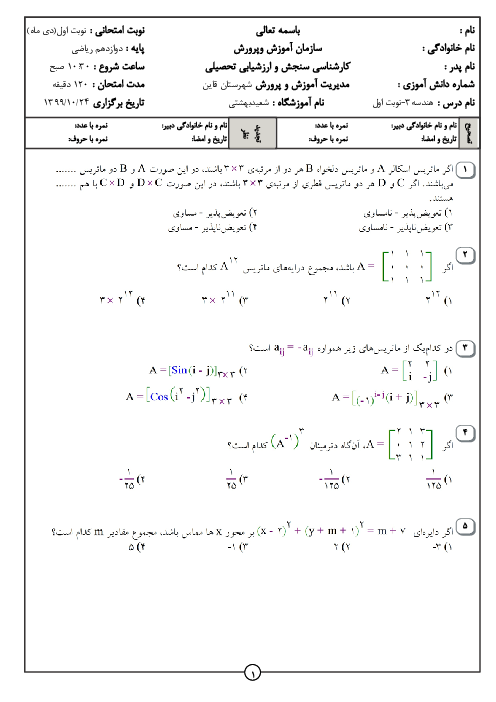
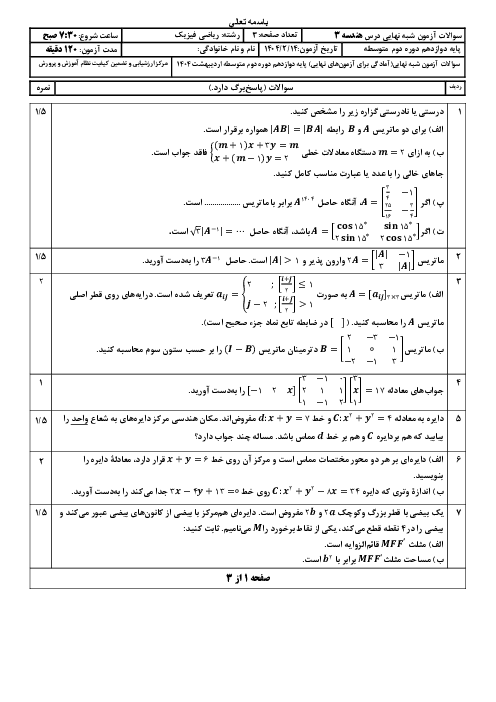
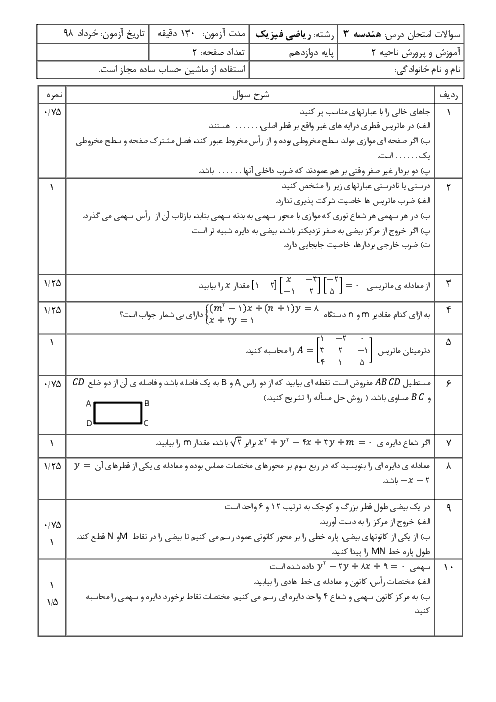
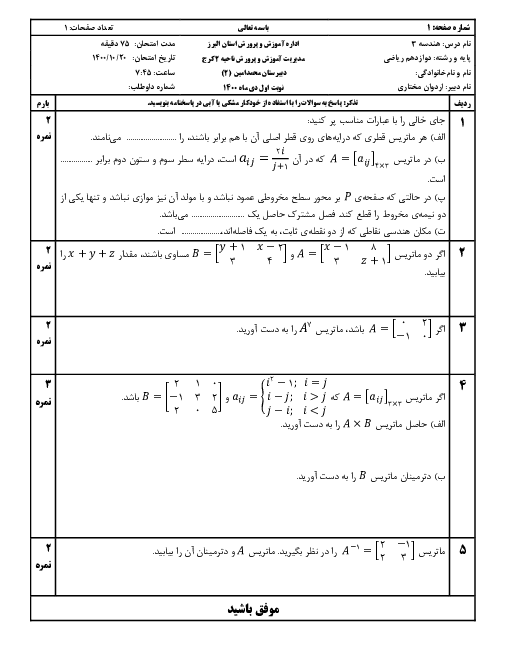
${{\left[ {{a}_{ij}} \right]}_{m\times n}}\pm {{\left[ {{b}_{ij}} \right]}_{m\times n}}={{\left[ {{a}_{ij}}\pm {{b}_{ij}} \right]}_{m\times n}}$

با جای‌گذاری مقادیر صفر، $\pi $ و $2\pi $ به‌جای $x$ در ماتریس $f(x)=\left[ \begin{matrix}
\begin{matrix}
\operatorname{Cos}\,x  \\
\operatorname{Sin}\,x  \\
\end{matrix} & \begin{matrix}
-\operatorname{Sin}\,x  \\
\operatorname{Cos}\,x  \\
\end{matrix}  \\
\end{matrix} \right]$ داریم:

$A=f(0)+f(\pi )+f(2\pi )=\left[ \begin{matrix}
\begin{matrix}
1  \\
0  \\
\end{matrix} & \begin{matrix}
0  \\
1  \\
\end{matrix}  \\
\end{matrix} \right]+\left[ \begin{matrix}
\begin{matrix}
-1  \\
0  \\
\end{matrix} & \begin{matrix}
0  \\
-1  \\
\end{matrix}  \\
\end{matrix} \right]+\left[ \begin{matrix}
\begin{matrix}
1  \\
0  \\
\end{matrix} & \begin{matrix}
0  \\
1  \\
\end{matrix}  \\
\end{matrix} \right]=\left[ \begin{matrix}
\begin{matrix}
1  \\
0  \\
\end{matrix} & \begin{matrix}
0  \\
1  \\
\end{matrix}  \\
\end{matrix} \right]$

بنابراین مجموع درایه‌های این ماتریس، برابر است با: $1+1=2$