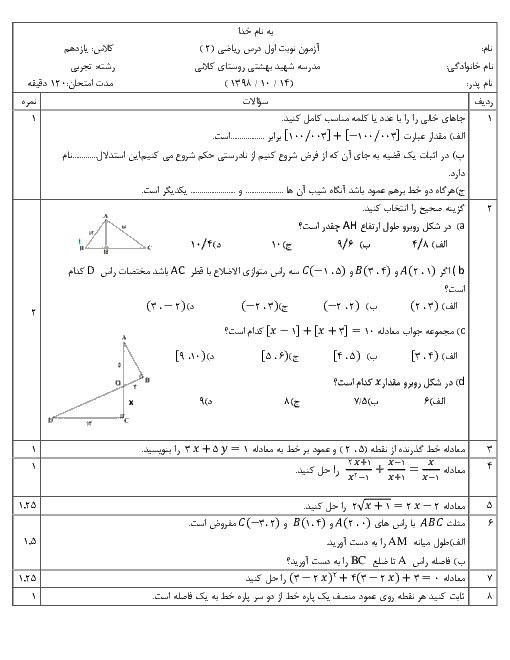
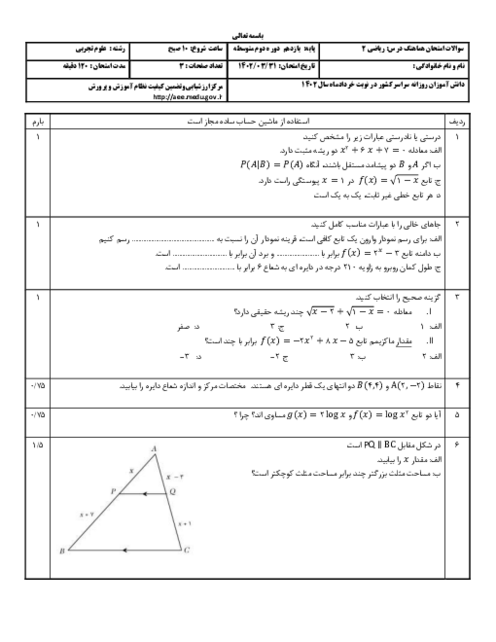
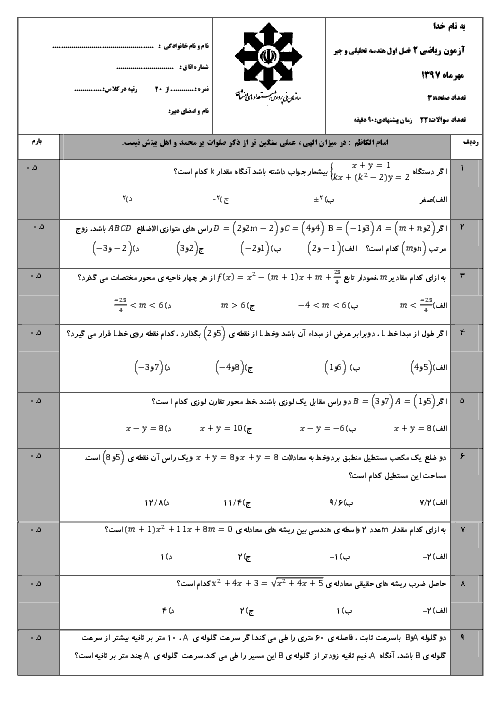
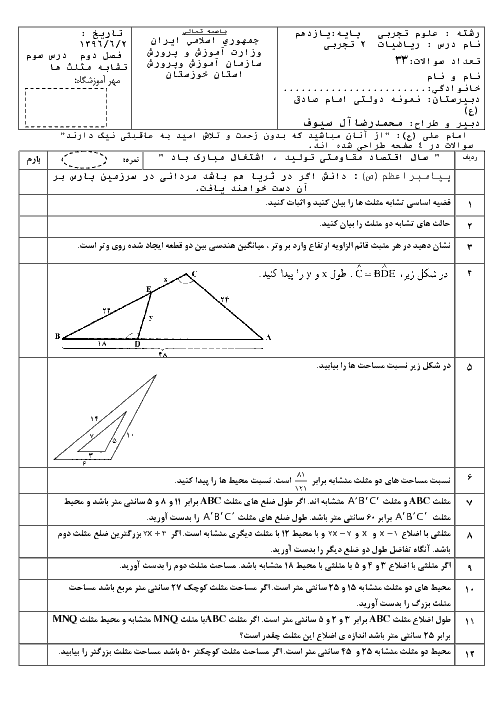
شیب خط Lبرابر منفی سه چهارم است پس شیب خط مطلوب که عمود بر آن است چهار سوم می شود.در نتیجه معادله ی آن به صورت 

$
{y}\mathrm{{=}}\frac{4}{3}{x}\mathrm{{+}}{n}
$ یا 3y-4x-3n=0 است. از طرفی فاصله نقطه (1,0) از این خط برابر 2 است ،پس داریم:

$
\frac{{\mathrm{|}}\mathrm{{-}}{4}\mathrm{{+}}{0}\mathrm{{-}}{3}{n}{\mathrm{|}}}{\sqrt{{\mathrm{16}}\mathrm{{+}}{9}}}\mathrm{{=}}{2}
$

$
\hspace{0.33em}{\mathrm{|}}\mathrm{{-}}{4}\mathrm{{-}}{3}{n}{\mathrm{|}}\mathrm{{=}}{\mathrm{10}}\mathrm{\Rightarrow}\mathrm{{-}}{4}\mathrm{{-}}{3}{n}\mathrm{{=}}\mathrm{\pm}{\mathrm{10}}
$

$
\hspace{0.33em}\mathrm{\Rightarrow}\mathrm{{-}}{3}{n}\mathrm{{=}}{\mathrm{10}}\mathrm{{+}}{4}\mathrm{\Rightarrow}{n}\mathrm{{=}}\mathrm{{-}}\frac{\mathrm{14}}{3}
$

$
\mathrm{{-}}{3}{n}\mathrm{{=}}\mathrm{{-}}{\mathrm{10}}\mathrm{{+}}{4}\mathrm{\Rightarrow}{n}\mathrm{{=}}{2}
$

$
\mathrm{\Rightarrow}{y}\mathrm{{=}}\frac{4}{3}{x}\mathrm{{-}}\frac{\mathrm{14}}{3}
$

یا 

$
\hspace{0.33em}{y}\mathrm{{=}}\frac{4}{3}{x}\mathrm{{+}}{2}
$