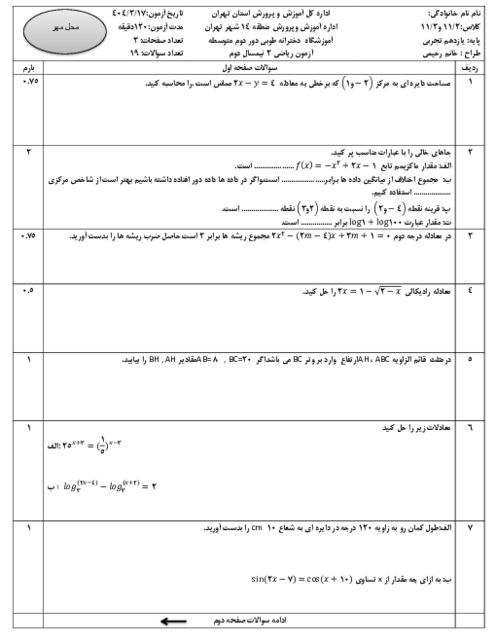
$\underset{x\to {{2}^{+}}}{\mathop{\lim }}\,\,f(x)=\underset{x\to {{2}^{+}}}{\mathop{\lim }}\,\,(3{{x}^{2}}-1)=3\times {{2}^{2}}-1=11$

$\underset{x\to {{2}^{-}}}{\mathop{\lim }}\,\,f(x)=\underset{x\to {{2}^{-}}}{\mathop{\lim }}\,\,(ax-b)=2a-b$

$\to 2a-b=11\,\,\,\,(1)$

$\underset{x\to -1}{\mathop{\lim }}\,\,f(x)=4\Rightarrow \underset{x\to -1}{\mathop{\lim }}\,\,(ax-b)=4\Rightarrow -a-b=4\Rightarrow a+b=-4\,\,\,\,(2)$

$\xrightarrow{(2)\,,\,(1)}\left\{ \begin{matrix}
2a-b=11  \\
a+b=-4  \\
\end{matrix} \right.\Rightarrow 3a=7\Rightarrow a=\frac{7}{3}$

$\xrightarrow{a+b=-4}\frac{7}{3}+b=-4\Rightarrow b=\frac{-19}{3}\Rightarrow a-b\Rightarrow \frac{7}{3}-(-\frac{19}{3})=\frac{26}{3}$