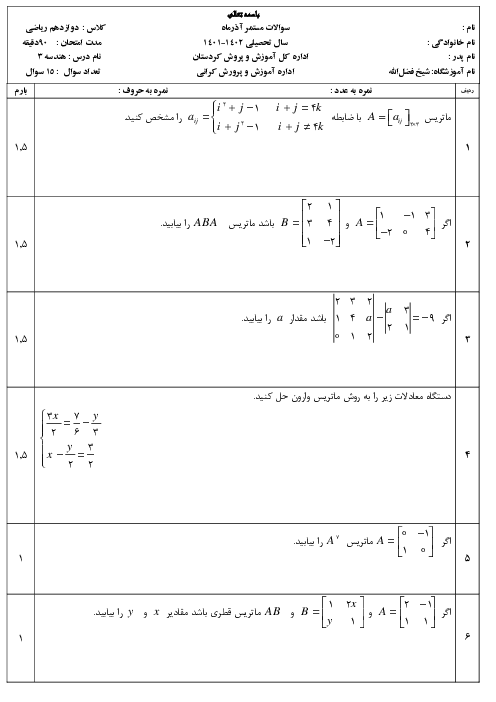
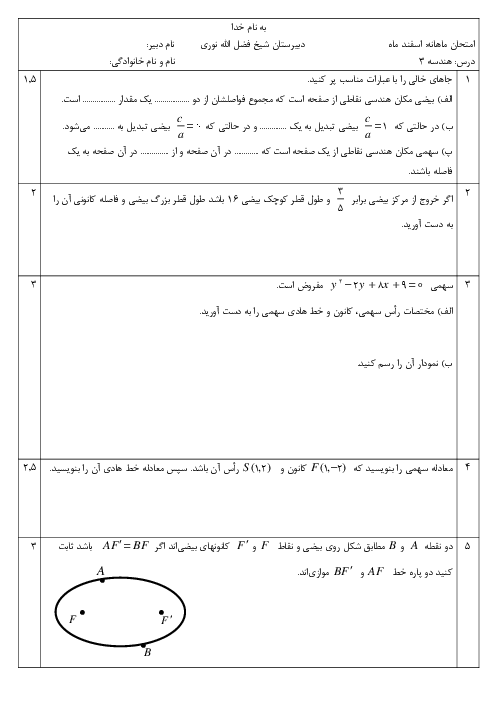
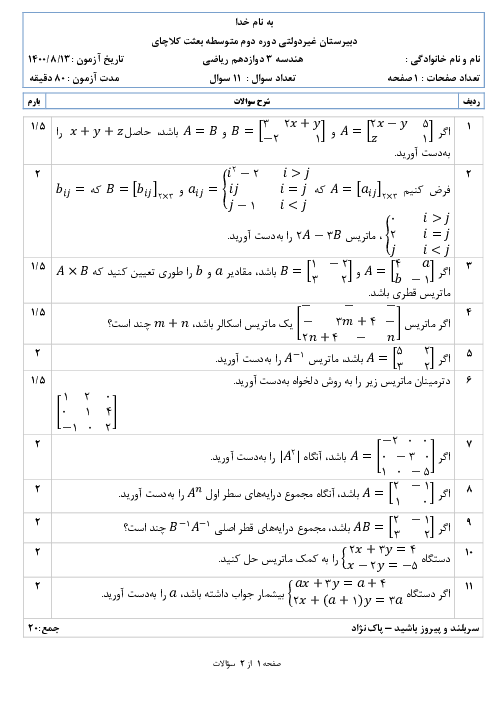
اگر $\overrightarrow{a}'$ تصویر بردار $a$ روی بردار $b$ باشد آن‌گاه قرینهٔ بردار $a$ نسبت به بردار $b$ از رابطهٔ $\overrightarrow{a}''=2\overrightarrow{a}'-\overrightarrow{a}$ به دست می‌آید. بنابراین:

$\overrightarrow{c}=\overrightarrow{a}''=2\overrightarrow{a}'-\overrightarrow{a}$

چون $\overrightarrow{a}.\overrightarrow{c}$ خواسته شده پس طرفین رابطهٔ بالا را در $\overrightarrow{a}$ ضرب می‌کنیم.

$\begin{align}  & \overrightarrow{a}.\overrightarrow{c}=\overrightarrow{a}.(2\overrightarrow{a}'-\overrightarrow{a})\Rightarrow \overrightarrow{a}.\overrightarrow{c}=2\overrightarrow{a}.\overrightarrow{a}'-\overrightarrow{a}.\overrightarrow{a} \\  & =2{{\left| \overrightarrow{a}' \right|}^{2}}-{{\left| \overrightarrow{a} \right|}^{2}}=2{{(\frac{\overrightarrow{a}.\overrightarrow{b}}{\left| \overrightarrow{b} \right|})}^{2}}-{{\left| \overrightarrow{a} \right|}^{2}} \\  & \Rightarrow \overrightarrow{a}.\overrightarrow{c}=2{{(\frac{1,-1,0).(2,-1,2)}{\sqrt{4+1+4}})}^{2}}-{{(\sqrt{1+1+0})}^{2}} \\  & \Rightarrow \overrightarrow{a}.\overrightarrow{c}=2{{(\frac{3}{3})}^{2}}-{{(\sqrt{2})}^{2}}=2-2=0 \\ \end{align}$