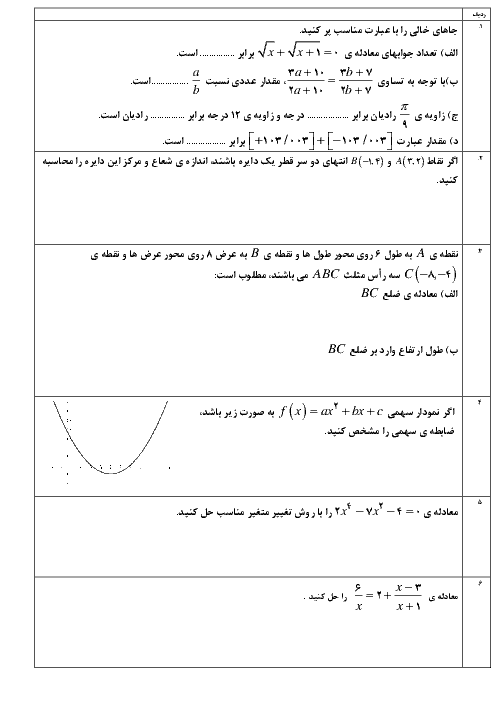
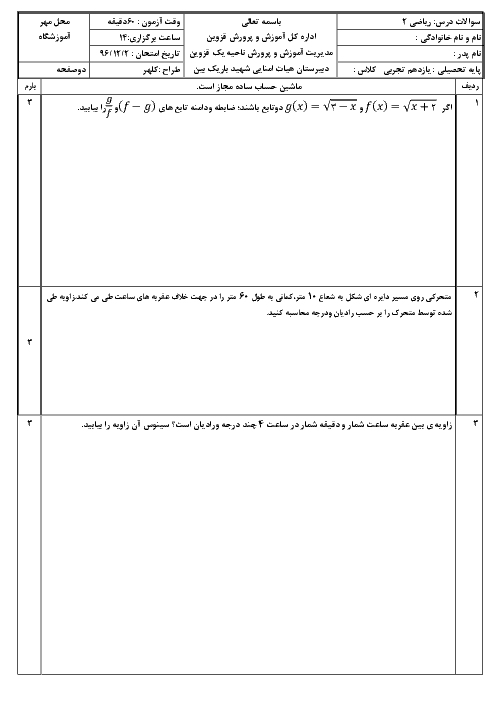
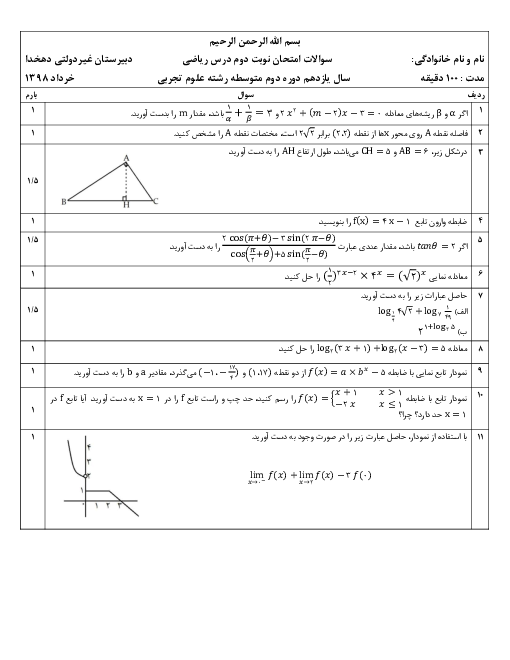
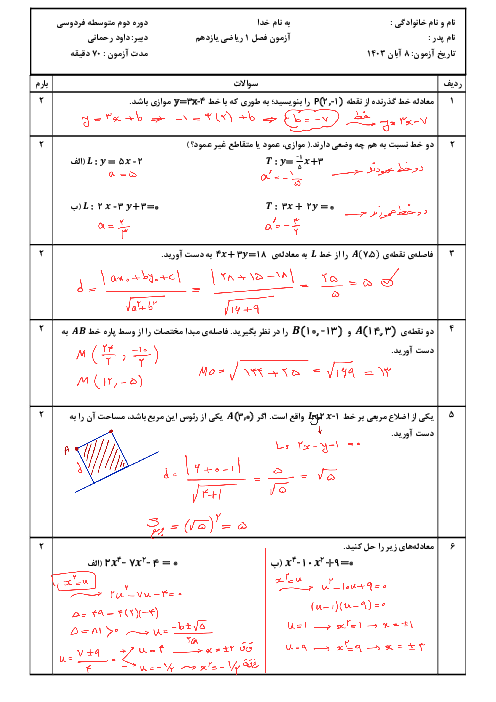اگر $f(x) = \left\{ \begin{gathered}
2x + 1\,\,\,\,\,,\,\,\,\,\,x \geqslant 1 \hfill \cr
3x - 2\,\,\,\,\,,\,\,\,\,\,x < 1 \hfill \cr
\end{gathered} \right.$ و $g(x) = \left\{ \begin{gathered}
3 - 2x\,\,\,\,\,,\,\,\,\,\,x \geqslant 1 \hfill \cr
5x + 3\,\,\,\,\,,\,\,\,\,\,x < 1 \hfill \cr
\end{gathered} \right.$ باشد توابع $f \pm g$ را محاسبه نمایید.
پاسخ تشریحی :
تحلیل ویدئویی تست
تحلیل ویدئویی برای این تست ثبت نشده است!
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...