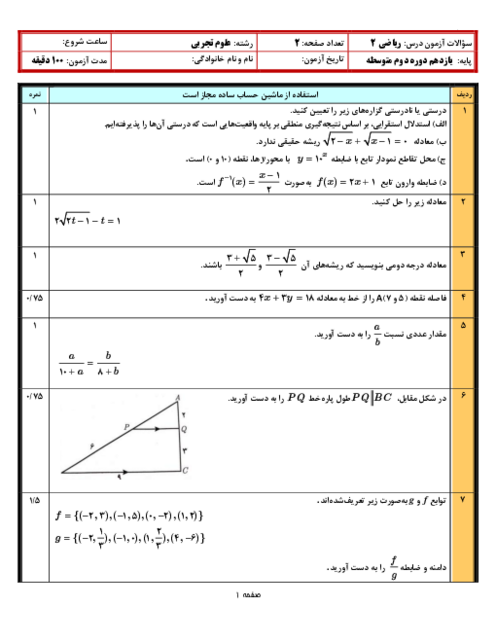
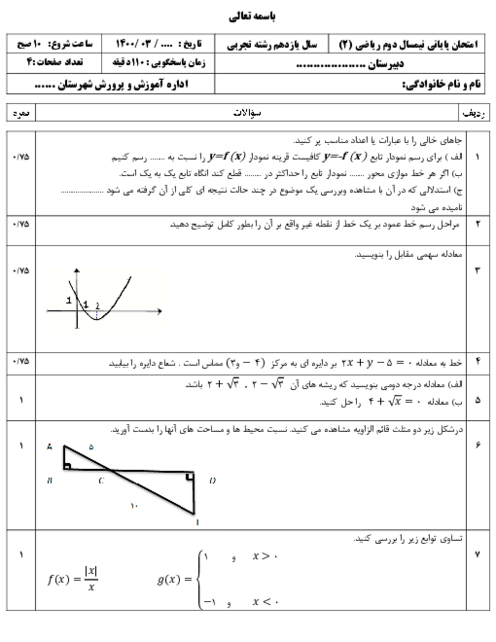
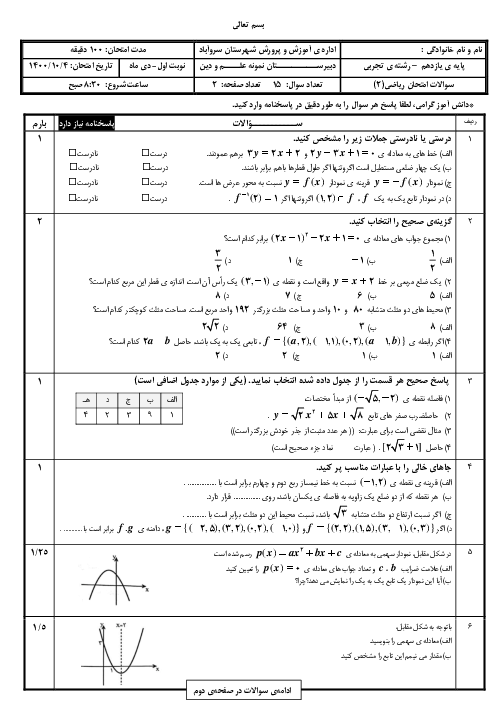
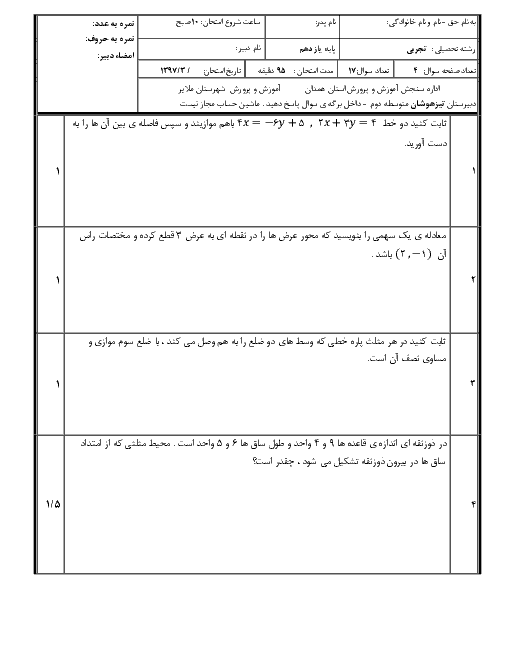
الف) B: حداقل دو مهره خارج شده سفید باشد

$n(B) = \left( \begin{gathered}  4 \hfill \\  2 \hfill \\ \end{gathered}  \right)\left( \begin{gathered}  8 \hfill \\
  1 \hfill \\ \end{gathered}  \right) + \left( \begin{gathered}  4 \hfill \\  3 \hfill \\ \end{gathered}  \right) = 6(8) + 4 = 52$

A: دقیقا دو مهره خارج شده سفید باشد.

$n(A \cap B) = \left( \begin{gathered}  4 \hfill \\  2 \hfill \\ \end{gathered}  \right)\left( \begin{gathered}  8 \hfill \\  1 \hfill \\ \end{gathered}  \right) = 6(8) = 48 \Rightarrow P(A|B) = \frac{{n(A \cap B)}}{{n(B)}} = \frac{{48}}{{52}} = \frac{{12}}{{13}}$

ب) B: سه مهره خارج شده همرنگ باشند.

$n(B) = \left( \begin{gathered}  4 \hfill \\  3 \hfill \\ \end{gathered}  \right) + \left( \begin{gathered}  5 \hfill \\  3 \hfill \\ \end{gathered}  \right) + \left( \begin{gathered}  3 \hfill \\  3 \hfill \\ \end{gathered}  \right) = 4 + 10 + 1 = 15$

A: هر سه مهره سفید باشند.

$n(A \cap B) = \left( \begin{gathered}  4 \hfill \\  3 \hfill \\ \end{gathered}  \right) = 4 \Rightarrow P(A|B) = \frac{{n(A \cap B)}}{{n(B)}} = \frac{4}{{15}}$

پ) B: دقیقاً یکی از مهره‌های خارج شده سیاه باشد.

$n(B) = \left( \begin{gathered}  5 \hfill \\  1 \hfill \\ \end{gathered}  \right)\left( \begin{gathered}  7 \hfill \\  2 \hfill \\ \end{gathered}  \right) = 5(21) = 105$

A: رنگ مهره‌ها متفاوت باشند.

$n(A \cap B) = \left( \begin{gathered}  4 \hfill \\  1 \hfill \\ \end{gathered}  \right)\left( \begin{gathered}  5 \hfill \\  1 \hfill \\ \end{gathered}  \right)\left( \begin{gathered}  3 \hfill \\  1 \hfill \\ \end{gathered}  \right) = 4(5)(3) = 60 \Rightarrow P(A|B) = \frac{{n(A \cap B)}}{{n(B)}} = \frac{{60}}{{105}} = \frac{4}{7}$