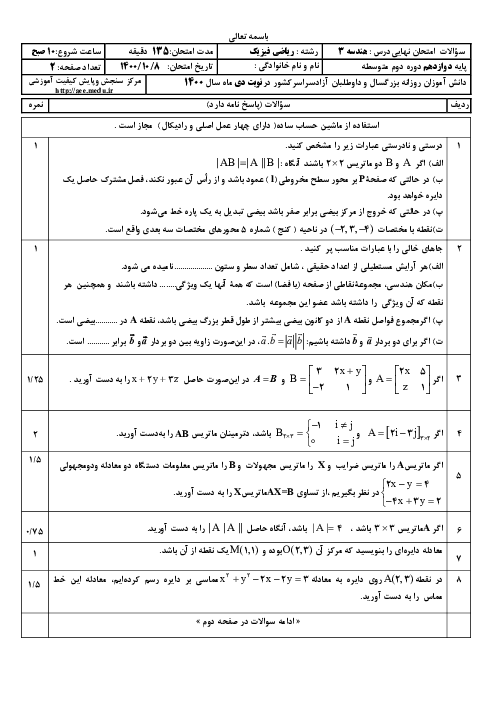
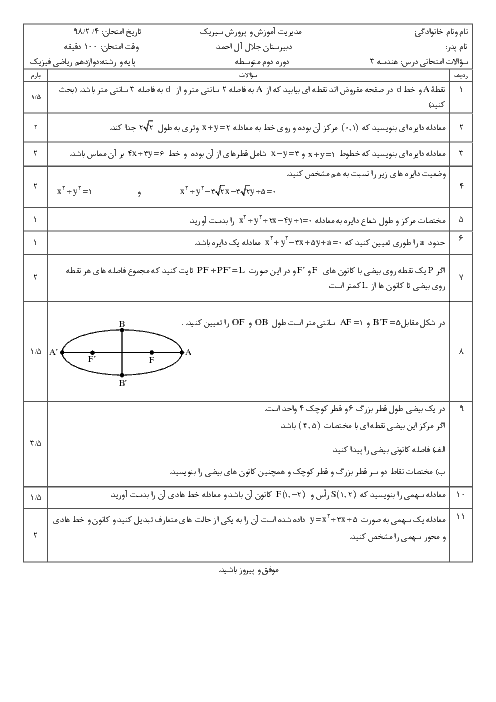
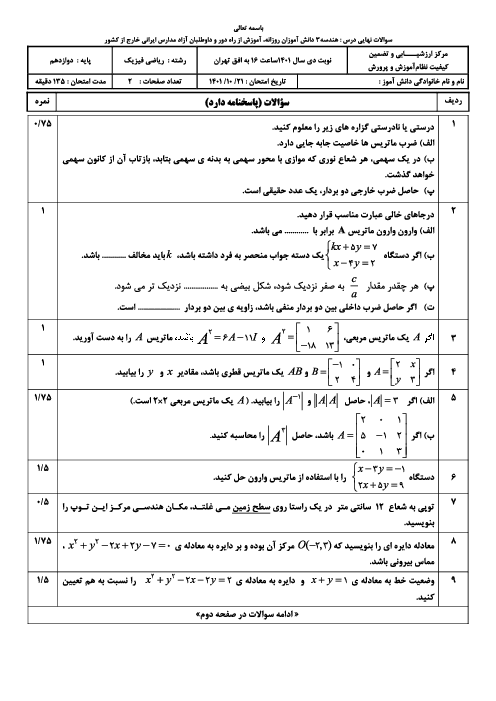
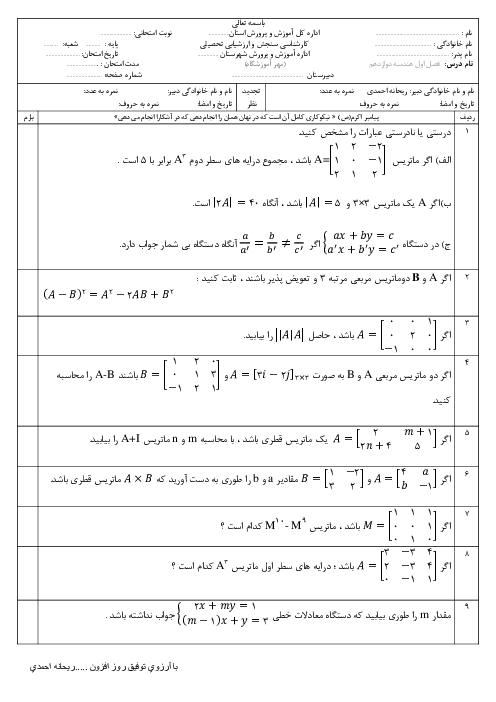
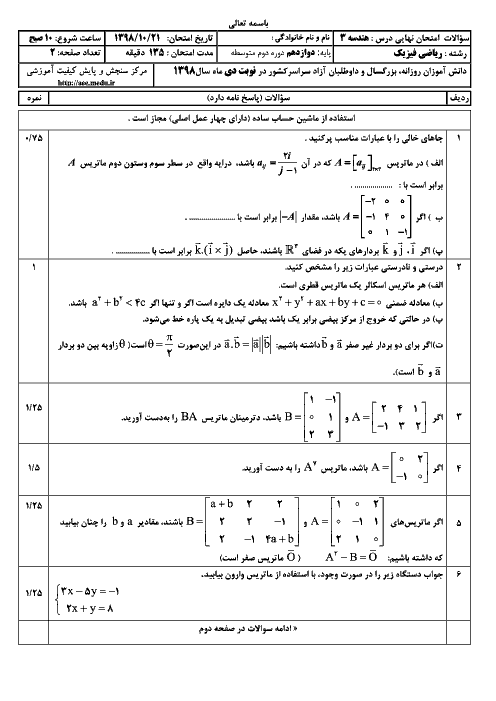
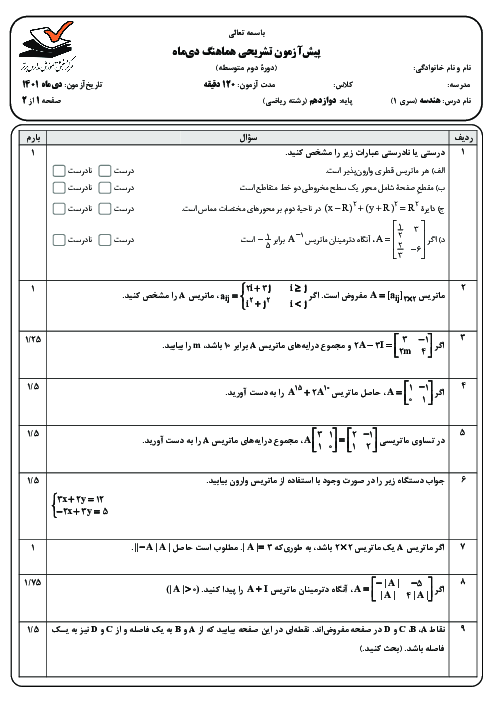
نکته: وارون ماتریس $A=\left[ \begin{matrix} \begin{matrix} a & b  \\ \end{matrix}  \\ \begin{matrix} c & d  \\ \end{matrix}  \\ \end{matrix} \right]$ با شرط $ad-bc\ne 0$، برابر است با: ${{A}^{-1}}=\frac{1}{ad-bc}\left[ \begin{matrix} \begin{matrix} d & -b  \\ \end{matrix}  \\ \begin{matrix} -c & a  \\ \end{matrix}  \\ \end{matrix} \right]$

ابتدا داریم:

$A+B=8AB\xrightarrow{{{A}^{-1}}\times }l+{{A}^{-1}}B=8B\xrightarrow{\times {{B}^{-1}}}{{B}^{-1}}+{{A}^{-1}}=8l(*)$

اكنون وارون ماتريس $A$ را به‌دست می‌آوريم: 

${{A}^{-1}}={{\left[ \begin{matrix} \begin{matrix} 5 & 3  \\ \end{matrix}  \\ \begin{matrix} 7 & 4  \\ \end{matrix}  \\ \end{matrix} \right]}^{-1}}=\frac{1}{20-21}\left[ \begin{matrix} \begin{matrix} 4 & -3  \\ \end{matrix}  \\ \begin{matrix} -7 & 5  \\ \end{matrix}  \\ \end{matrix} \right]=\left[ \begin{matrix} \begin{matrix} -4 & 3  \\ \end{matrix}  \\ \begin{matrix} 7 & -5  \\ \end{matrix}  \\ \end{matrix} \right]$

با جای‌گذاری در (*) داریم:

${{B}^{-1}}+{{A}^{-1}}=8l\Rightarrow {{B}^{-1}}+\left[ \begin{matrix} \begin{matrix} -4 & 3  \\ \end{matrix}  \\ \begin{matrix} 7 & -5  \\ \end{matrix}  \\ \end{matrix} \right]=\left[ \begin{matrix} \begin{matrix} 8 & 0  \\ \end{matrix}  \\ \begin{matrix} 0 & 8  \\ \end{matrix}  \\ \end{matrix} \right]\Rightarrow $${{B}^{-1}}=\left[ \begin{matrix} \begin{matrix} 12 & -3  \\ \end{matrix}  \\ \begin{matrix} -7 & 13  \\ \end{matrix}  \\ \end{matrix} \right]$

بنابراين مجموع درايه‌های ماتريس ${{B}^{-1}}$ برابر 15 است.