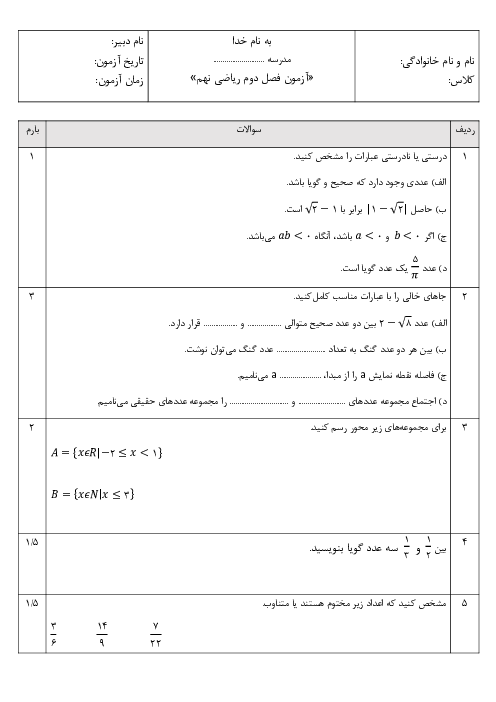
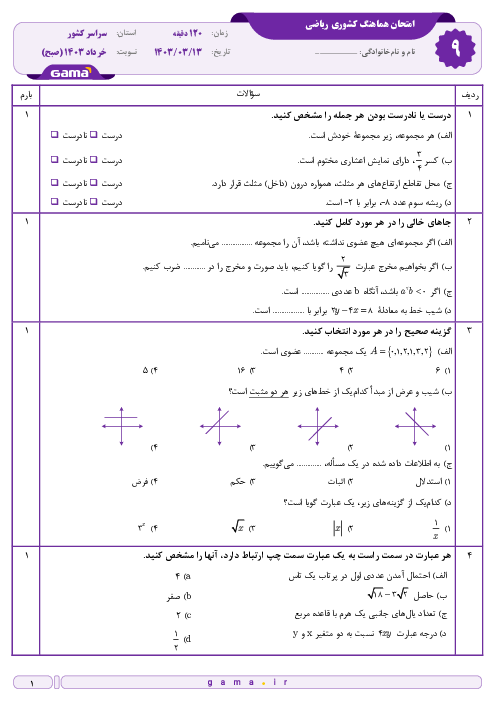
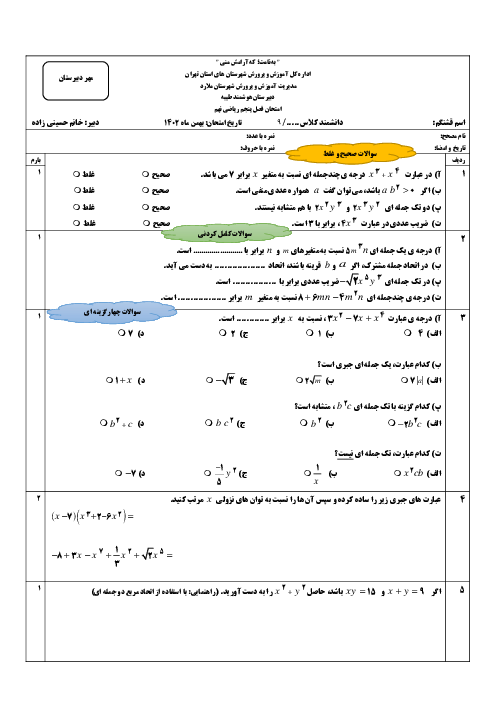
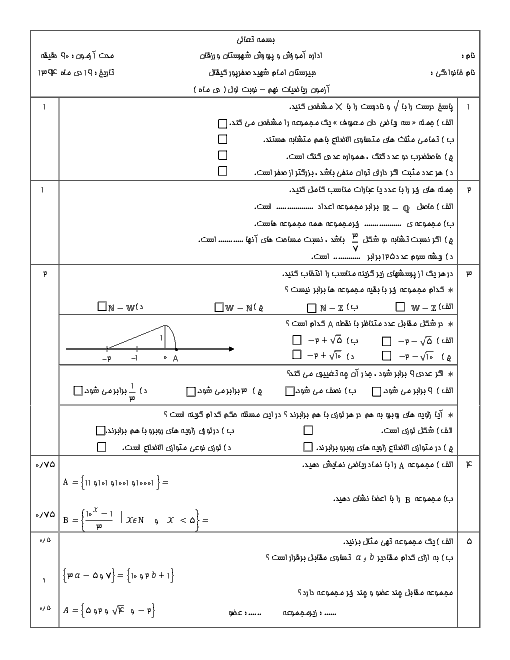
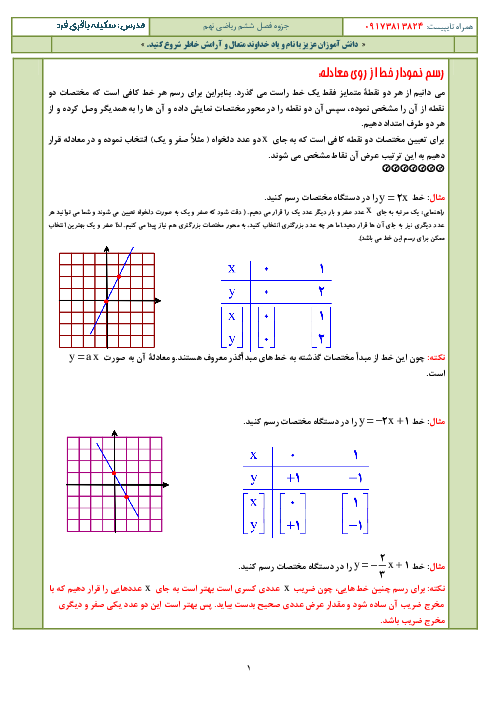
$\mathop {({{ - 1} \over 3})}\nolimits^4 \mathop {({9 \over 2})}\nolimits^{ - 5} \mathop {({9 \over 4})}\nolimits^2  = {1 \over {\mathop 3\nolimits^4 }} \times \mathop {({2 \over 9})}\nolimits^5  \times \mathop {({9 \over 4})}\nolimits^2  = {1 \over {\mathop 3\nolimits^4 }} \times {{\mathop 2\nolimits^5 } \over {\mathop 9\nolimits^5 }} \times {{\mathop 9\nolimits^2 } \over {\mathop 4\nolimits^2 }} = {1 \over {\mathop 3\nolimits^4 }} \times {{\mathop 2\nolimits^5 } \over {\mathop {(\mathop 3\nolimits^2 )}\nolimits^5 }} \times {{\mathop {\left( {\mathop 3\nolimits^2 } \right)}\nolimits^2 } \over {\mathop {\left( {\mathop 2\nolimits^2 } \right)}\nolimits^2 }} = {1 \over {\mathop 3\nolimits^4 }} \times {{\mathop 2\nolimits^5 } \over {\mathop 3\nolimits^{10} }} \times {{\mathop 3\nolimits^4 } \over {\mathop 2\nolimits^4 }} = {{\mathop 2\nolimits^{5 - 4} } \over {\mathop 3\nolimits^{10} }} = {2 \over {\mathop 3\nolimits^{10} }}$