درس 3: روابط بین نسبت‌های مثلثاتی
ریاضی (1)
دهم
دوره دوم متوسطه- نظری
علوم تجربی
درسنامه آموزشی این مبحث
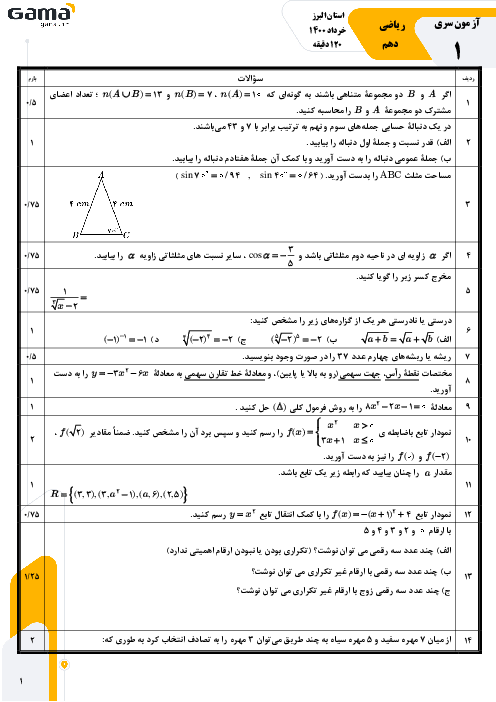
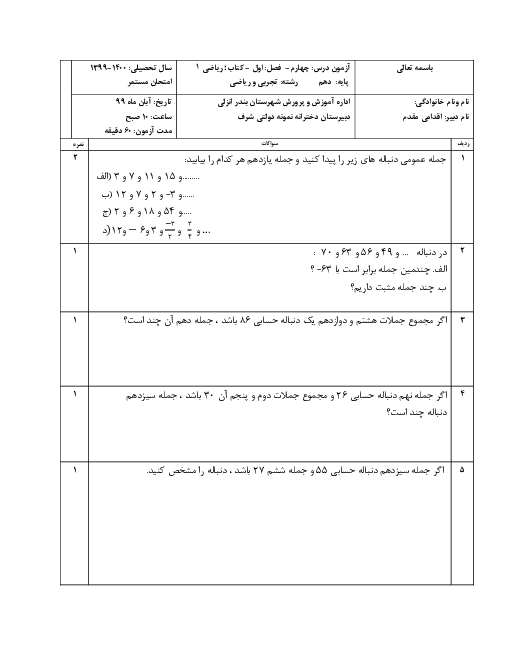
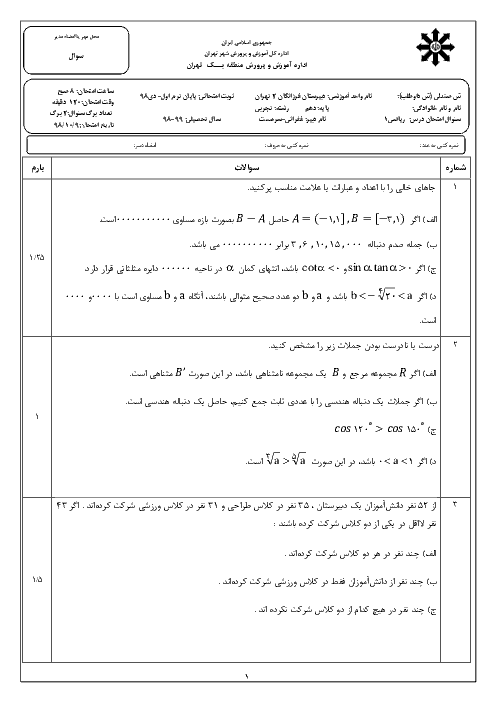
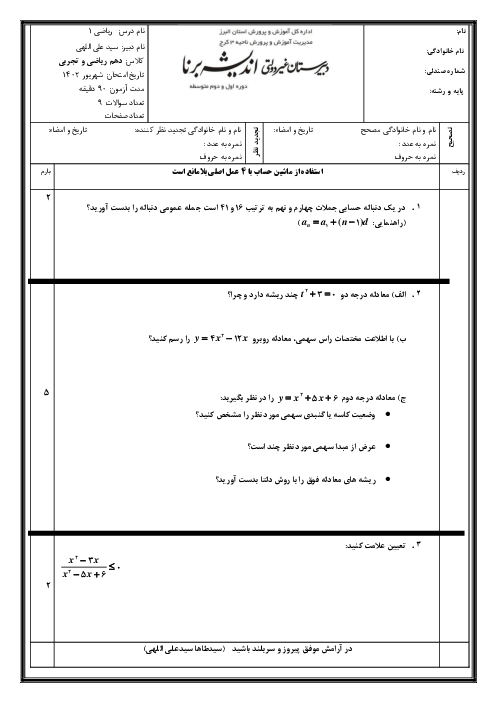
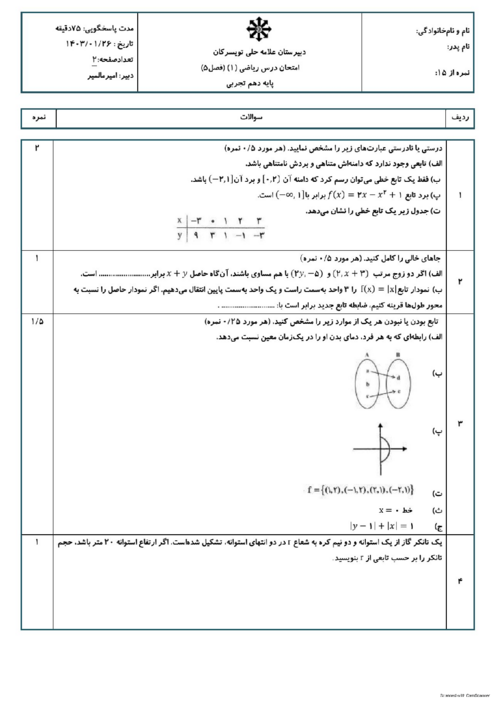
کدام تساوی در مورد روابط بین نسبت‌های مثلثاتی درست نیست؟
1 )
$ (1 + tan\theta)(1 + cot\theta) - \frac{1}{sin\theta cos\theta} = 2$
$ (1 + tan\theta)(1 + cot\theta) = \frac{1}{sin\theta cos\theta}$
3 )
$ tan^2\theta - sin^2\theta = tan^2\theta sin^2\theta$
4 )
$ cos^4\theta - sin^4\theta = cos^2\theta - sin^2\theta$
پاسخ تشریحی :
تحلیل ویدئویی تست
امیرحسین مزدور 
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...