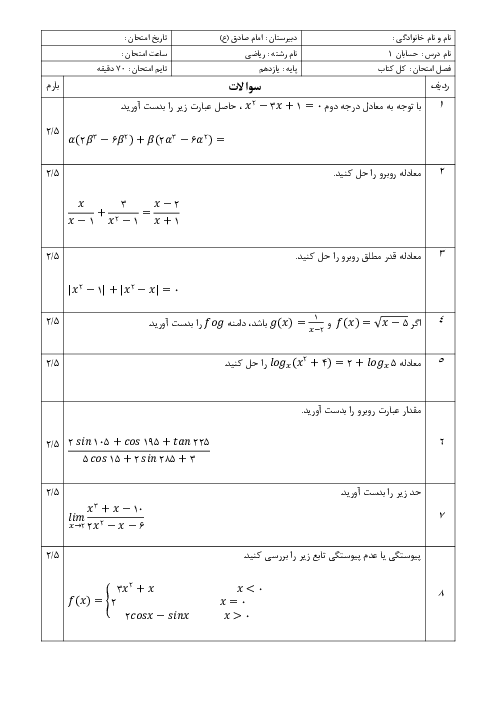
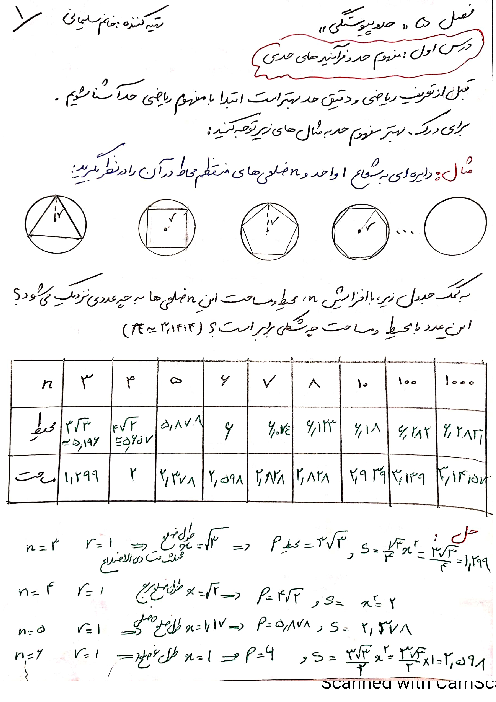
$\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,f(x)=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,\frac{2{{x}^{2}}-3x+1}{{{x}^{2}}-1}=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,\frac{(x-1)(2x-1)}{(x-1)(x+1)}=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,\frac{2x-1}{x+1}=\frac{1}{2}$
$\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,f(x)=\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,\sqrt{5{{x}^{2}}-a}=\sqrt{5-a}$
$f$ در $x=1$ حد دارد، بنابراین:
$\sqrt{5-a}=\frac{1}{2}\Rightarrow 5-a=\frac{1}{4}\Rightarrow a=5-\frac{1}{4}=\frac{19}{4}$
$\underset{x\to {{(-2)}^{+}}}{\mathop{\lim }}\,f(x)=\underset{x\to {{(-2)}^{+}}}{\mathop{\lim }}\,\frac{2{{x}^{2}}-3x+1}{{{x}^{2}}-1}=\frac{15}{3}=5$
$\underset{x\to {{(-2)}^{-}}}{\mathop{\lim }}\,f(x)=\underset{x\to {{(-2)}^{-}}}{\mathop{\lim }}\,b\left[ x \right]+\frac{\left| x+2 \right|}{x+2}=-3b-1$
$f$ در $x=-2$ حد دارد، بنابراین:
$\Rightarrow -3b-1=5\Rightarrow -3b=6\Rightarrow b=-2$
$\Rightarrow 2a\times b=2\times \frac{19}{4}\times (-2)=-19$