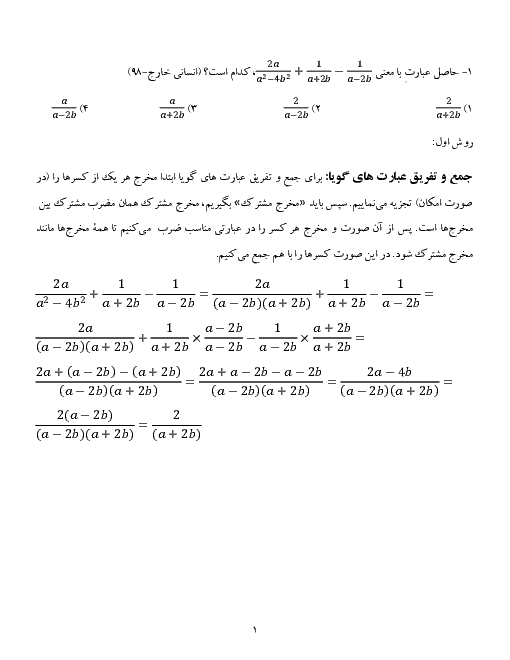
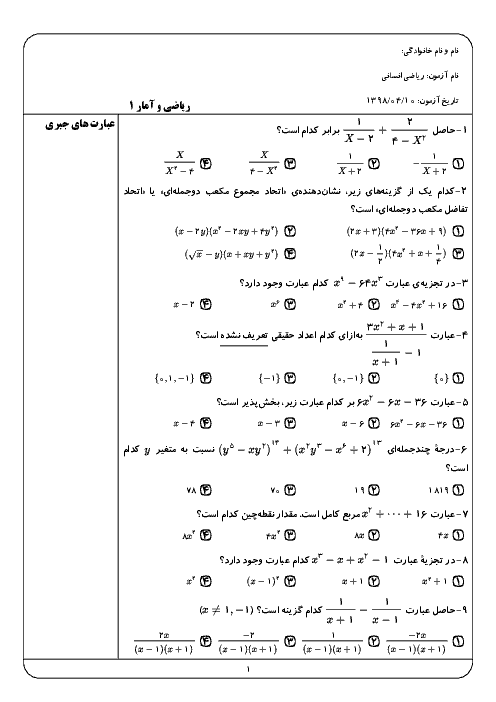
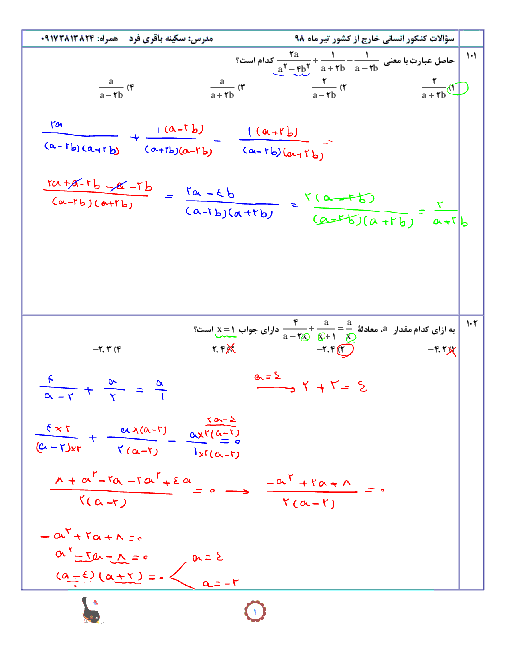
$n(s) = \left( \begin{gathered}
7 \hfill \\
2 \hfill \\
\end{gathered} \right) = \frac{{7 \times 6}}{2} = 21$
$n(A) = \left( \begin{gathered}
2 \hfill \\
2 \hfill \\
\end{gathered} \right) \times \left( \begin{gathered}
3 \hfill \\
3 \hfill \\
\end{gathered} \right) $
$+ \left( \begin{gathered}
2 \hfill \\
1 \hfill \\
\end{gathered} \right) \times \left( \begin{gathered}
2 \hfill \\
1 \hfill \\
\end{gathered} \right) \times \left( \begin{gathered}
3 \hfill \\
3 \hfill \\
\end{gathered} \right) $
$+ \left( \begin{gathered}
2 \hfill \\
2 \hfill \\
\end{gathered} \right) \times \left( \begin{gathered}
2 \hfill \\
1 \hfill \\
\end{gathered} \right) \times \left( \begin{gathered}
3 \hfill \\
2 \hfill \\
\end{gathered} \right)$
$ = 1 + 4 + 6 = 11$
$ \Rightarrow p(A) = \frac{{11}}{{21}}$