نکته:  $ac\overset{m}{\mathop{\equiv }}\,bc\Rightarrow a\overset{\frac{m}{(m,c)}}{\mathop{\equiv }}\,b$ 

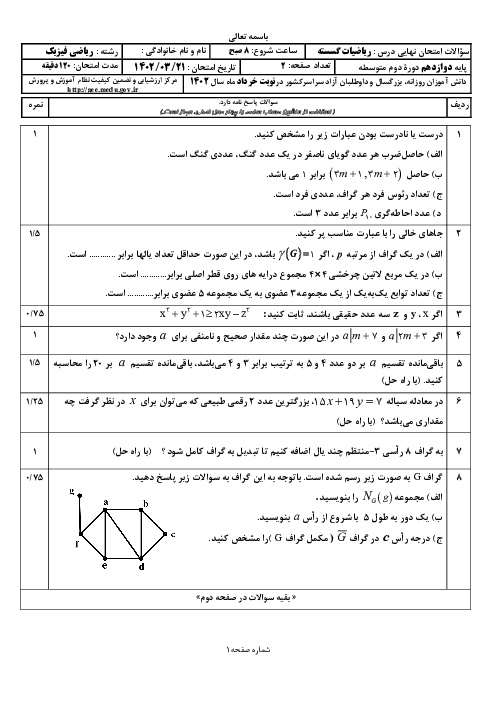
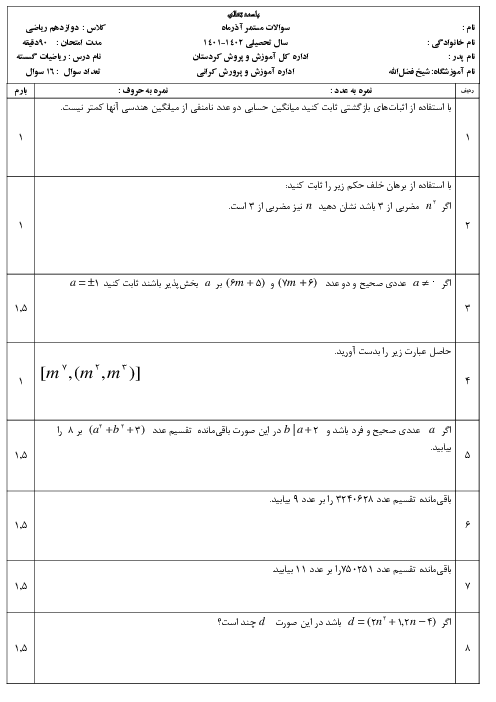
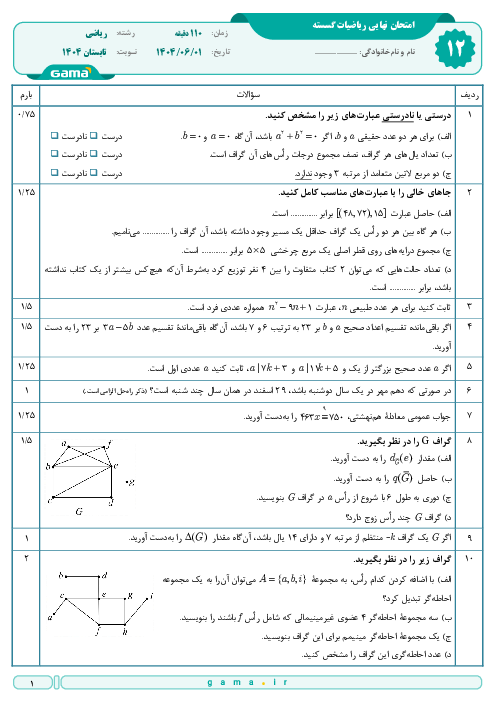
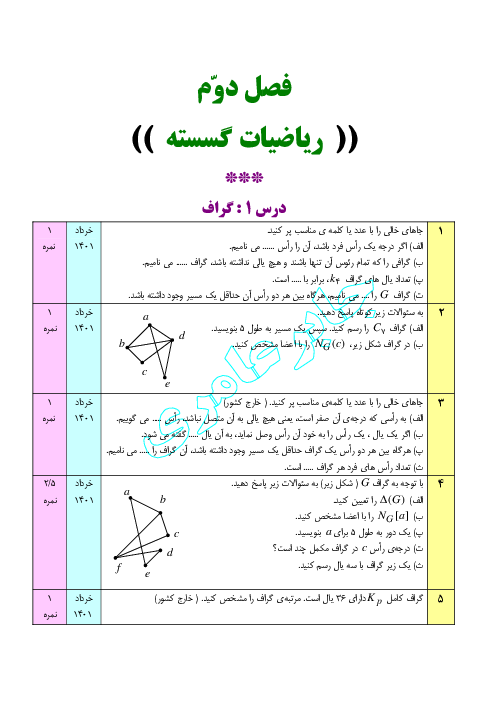
نکته: می‌توان به یک طرف یا هر دو طرف یک رابطه‌ی هم‌نهشتی، مضربی از پیمانه را اضافه کرد:

$a\overset{m}{\mathop{\equiv }}\,b\Rightarrow a\overset{m}{\mathop{\equiv }}\,mk+b,a+mk\overset{m}{\mathop{\equiv }}\,b,a+mk\overset{m}{\mathop{\equiv }}\,b+m{k}'$

نکته: $a\left| b \right.\Rightarrow a\left| mb \right.(m\in Z)$ 

فرض کنیم $(5n-2,7n+3)=d$. در این صورت داریم:

$\left\{ _{d\left| 7n+3 \right.\xrightarrow{\times 5}d\left| 35n+115 \right.}^{d\left| 5n-2 \right.\xrightarrow{\times 7}d\left| 35n-14 \right.} \right.\xrightarrow{-}d\left| 29 \right.\Rightarrow d=1,29$  

برای اینکه این دو عدد نسبت به هم اول نباشند، باید داشته باشیم  $d=29$. به‌ازای این مقدار داریم: 

$29\left| 5n-2 \right.\Rightarrow 5n-2\overset{29}{\mathop{\equiv }}\,0\Rightarrow 5n\overset{29}{\mathop{\equiv }}\,2\Rightarrow 5n\overset{29}{\mathop{\equiv }}\,2+29\times 2$

$\Rightarrow 5n\overset{29}{\mathop{\equiv }}\,60\xrightarrow[(29,5)=1]{\div 5}n\overset{29}{\mathop{\equiv }}\,12\Rightarrow n=29q+12$ 

به‌ازای $q=0,1,2,3$، عدد طبیعی  $n$ دو رقمی می‌شود، پس $4$ جواب داریم.